 Don't call me Shirley.
Don't call me Shirley.
Welcome to my cubicle! Here is what I do
all day.
A little while back --by which I mean over a year
ago-- my supervisor looked at a plot that I made, wrinkled his nose, and said,
"That looks like some sort of parametric resonance, doesn't it? Surely that
makes sense, and it should be easy to show on paper why that happens.
[pause...] Don't you agree? [pause...] Do you know what parametric resonance
is?"
yeah.. Every time that man says the word "surely", I know I'm in trouble. Months and months and months later, and after a million to-do lists where the line "Figure out param. res. shit" was left un- crossed out, I know this much:
I know that you get PR when you have a differential equation that looks like this:
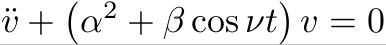
I know that the problem that I've been staring at for nigh 3 and a half years produces an equation like this:

The similarity is that, in the first equation, one of the parameters is a periodic function (the cosine function), whereas in the second equation, that expression in brackets has a several periodic components to it (there are cosine functions, but more importantly, that x-bar term oscillates in time).
In the first equation, the magic happens if the frequency of the parameter (\nu) is an integer multiple of the natural frequency (\alpha) of the system -- the solution grows quickly because of parametric resonance. It only works well (whatever that means) if this integer multiple is 1 or 2 (and 2 is better). You can solve that equation numerically, and it looks like this:
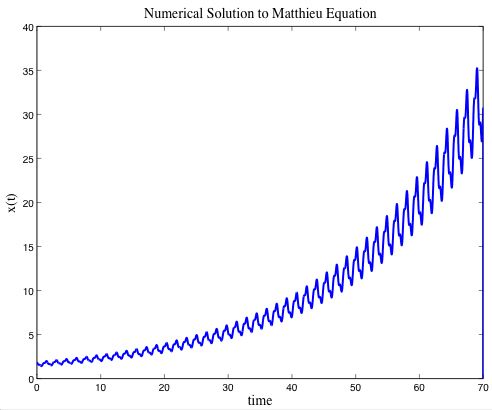
Whoo, exponential growth!
In the second equation, it just so happens that the frequency of the periodic parameter (x-bar) is an integer multiple of the natural frequency (\epsilon^-1), in fact, they are the same. This means that the integer multiple is 1, which is almost as good as 2.
So, if you wave your hands around in the air a bit and mutter some shit about asymptotic limits, the second equation should also have crazy growing solutions that are totally explainable, thus enabling me to write another paper, graduate earlier, and move to Rome to become a superstar at the European Space Agency and ride a Vespa to work every day and drink wine in the cafeteria and take romantic walks past the Colliseum.
Unfortunately the second equation has a whole lot of other shit in it (the C's and phi's), which makes it kind of an ugly cousin of the first equation. If you take all that shit out and integrate numerically, you will get this:
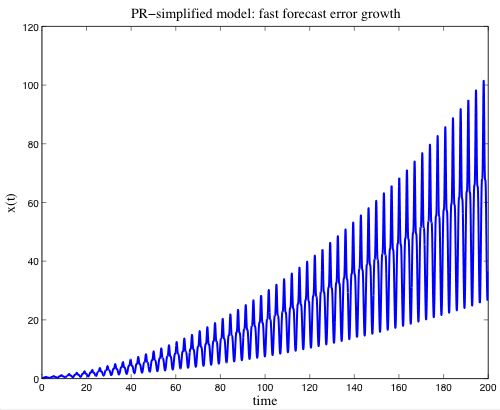
Mmmhmm, that smells like PR to me. Woot.
But it turns out all that other shit is kind of important, so you might want to stick it back in. Integrate that mofo, and you get this:
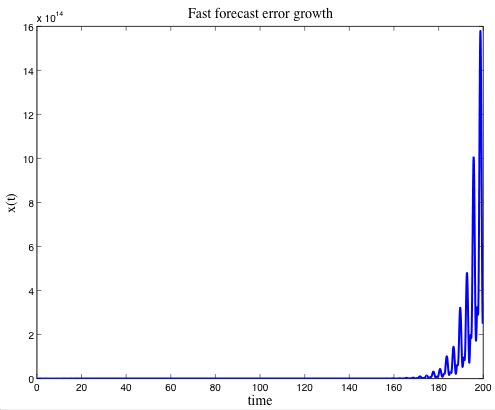
Yaaa!! Could this revolutionize the world of atmospheric data assimilation?? Maybe. Except that if you change your initial conditions a wee bit, you get this:

Do you see the exponential growth? I sure don't. I see a bunch of shit that lost its connection to physical reality a long, long time ago. What the crap gives?? While writing this whole thing out has made me feel pretty smart, I still don't have an answer to the bossman. I want a beer.
yeah.. Every time that man says the word "surely", I know I'm in trouble. Months and months and months later, and after a million to-do lists where the line "Figure out param. res. shit" was left un- crossed out, I know this much:
I know that you get PR when you have a differential equation that looks like this:
I know that the problem that I've been staring at for nigh 3 and a half years produces an equation like this:
The similarity is that, in the first equation, one of the parameters is a periodic function (the cosine function), whereas in the second equation, that expression in brackets has a several periodic components to it (there are cosine functions, but more importantly, that x-bar term oscillates in time).
In the first equation, the magic happens if the frequency of the parameter (\nu) is an integer multiple of the natural frequency (\alpha) of the system -- the solution grows quickly because of parametric resonance. It only works well (whatever that means) if this integer multiple is 1 or 2 (and 2 is better). You can solve that equation numerically, and it looks like this:

Whoo, exponential growth!
In the second equation, it just so happens that the frequency of the periodic parameter (x-bar) is an integer multiple of the natural frequency (\epsilon^-1), in fact, they are the same. This means that the integer multiple is 1, which is almost as good as 2.
So, if you wave your hands around in the air a bit and mutter some shit about asymptotic limits, the second equation should also have crazy growing solutions that are totally explainable, thus enabling me to write another paper, graduate earlier, and move to Rome to become a superstar at the European Space Agency and ride a Vespa to work every day and drink wine in the cafeteria and take romantic walks past the Colliseum.
Unfortunately the second equation has a whole lot of other shit in it (the C's and phi's), which makes it kind of an ugly cousin of the first equation. If you take all that shit out and integrate numerically, you will get this:

Mmmhmm, that smells like PR to me. Woot.
But it turns out all that other shit is kind of important, so you might want to stick it back in. Integrate that mofo, and you get this:

Yaaa!! Could this revolutionize the world of atmospheric data assimilation?? Maybe. Except that if you change your initial conditions a wee bit, you get this:

Do you see the exponential growth? I sure don't. I see a bunch of shit that lost its connection to physical reality a long, long time ago. What the crap gives?? While writing this whole thing out has made me feel pretty smart, I still don't have an answer to the bossman. I want a beer.
Posted: Sat - July 30, 2005 at 12:00 PM
Quick Links
das bin ich:
german-born, american raised, canada-loving aspiring scientist / cat lady.

Categories
currently reading

If on a Winter's Night a Traveler by Italo Calvino

A Fine Balance by Rohinton Mistry
blogs I read
mostly random but fun shit:
hey, blog rings!

Previous | Next
Archives
Calendar
| Sun | Mon | Tue | Wed | Thu | Fri | Sat |
Statistics
Total entries in this blog:
Total entries in this category:
Published On: Jul 30, 2005 03:50 PM
Total entries in this category:
Published On: Jul 30, 2005 03:50 PM

